The Kind-Hearted Magician -- Guest Post by Kip Hansen
We have had a lot of seriousness over the last year. Covid Madness, possibly wonky elections, riots of the Left and riots of the Right, petty criminals become heroes and heroes are characterized as criminals. “Enough!” I say. Time for some intellectual amusement.
When I was a youth, and continuing into my late teens and early 20s, I was a semi-professional stage magician. That is, I was occasionally paid to perform on stage doing magic – it was only a hobby and I never earned anything like a living at it. But in the run up to my semi-professional career, I studied every book I could find -- buy, beg or borrow -- on the performance of stage magic, illusions, card tricks and the lives of the great magicians of the 19th and early 20th century. Sadly, I did not have the patience to learn coin manipulation tricks (see prestidigitation).
My real strength was the art of misdirection – shifting the attention of the audience away from my manipulation of the objects necessary to carry off the trick. I used an example of one of the techniques in an essay on climate science many years ago: The Magician's 'Red Scarf Trick'.
Stage magic, and stage illusions, all rely on the same basic underlying approach in order to make something impossible occur – to make magic. The Magician tells the audience he is doing one thing, the Magician lets the audience see that one thing, but all the while the Magician actually does something else altogether and the results, as far as the audience is concerned, are magical. That requires misdirection, both verbal and physical, and a great deal of sleight of hand.
Today I write about something that is very akin to “sleight of hand” – which we can think of as sleight of mind in which the Magician makes the audience (even if just an audience of one) think one thing when something else is the reality.
Our kind-hearted magician, The Magnificent Sam, is a little short of cash, so, sort of like the famous Penn and Teller, sets up a act in which he can earn some money*. It goes like this:
Sam invites a couple from the audience, Mr. and Mrs. Dupe, to the stage. Sam shows us all three cards, two Jokers and one Ace, then lays them on the table, face down and swirls them around a lot, mixing them up.
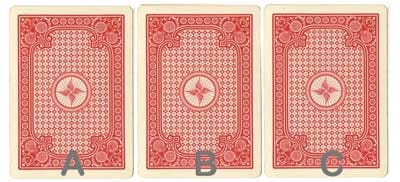
Sam then tells the Dupes that he will pay them $10 if they can “find the Ace”, and if they fail, they pay him $10. (Sounds a lot like street-side Three Card Monte doesn’t it?) The Dupes pick the middle card and the Magician puts a little Yellow Star sticker on it, but the Magician doesn’t turn it over yet.

You see, the newlywed Dupes are so young, so cute and needy looking, that the Magician takes pity on them and says, “Look, this card is a Joker”, and turns over the card on the left, leaving two cards – the one the Dupes picked and one other. Sam asks “Now, do you want to stick to your choice – card B? Or switch to the other one, card C?
Remember Mr. and Mrs. Dupe are playing only this once.
Questions:
Q1. Does the Magician’s revealing of one of the two Jokers improve the couple’s chance of winning? Does the Magician’s allowing them to pick again improve the couple’s chance of winning?
Q2. Regardless of your answer to Q1, what would you advise our young couple to do? Stick with their first choice, switch their choice, or flip a coin to pick between the two remaining cards?
Now, our Kind-hearted Magician, The Magnificent Sam, says “I can’t bring myself to cheat such a nice young couple. When you first picked, you had only a one-in-three chance of being right and winning $10. I’d like to improve your odds to make it fair. Here’s what I’ll do, using my Magic Wand as an Event Eraser, I’ll wave it over the cards like this . . . (waving wand slowly over the three cards now on the table) . . . and erase the last few minutes of time, when you were picking from three cards.”
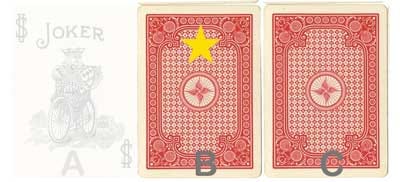
Sam waves the Magic Wand and lo-and-behold the Joker on the left, in position A, begins to fade from sight leaving just a little shadow. “That’s better,” Sam says, “now, would you like to move your Yellow Star sticker to card C or leave it on card B?” If your star is on the Ace, then you win $10, and if on the Joker, you pay me $10.”
Remember Mr. and Mrs. Dupe are playing only this once.
Question:
Q3. What are the odds – the probabilities – of the Dupes winning now? Do the odds change if they change their pick?
Take your time and answer the questions in the comments below. I think it will be more interesting if the questions are answered before we read on.
Many of you will have recognized the problem posed here as the classic Monty Hall Problem – alternately named the Monty Hall Dilemma. Marilyn vos Savant famously answered the question in her column in Parade in 1990 [ wiki ] resulting in a wide-ranging controversy in the mathematics and statistics worlds. [Here is Briggs's classic take on the MH Problem.]
Marilyn vos Savant answers, quite correctly, that in our Q2, the Dupes will double their chances of winning if they switch their choice. The number of proofs of this is nearly endless and can be found in the Wiki link.
However, Sam the Kind-hearted Magician gives us another look at the problem. He uses magic to erase the original circumstances of their choice, which was to pick one of three cards (or doors in the original TV show). Having “erased” that event left the Dupes with exactly the same situation as they found themselves when we asked Q1 and Q2.
Exactly? Yes, precisely that.
Let’s check carefully, comparing the scenarios presented by vos Savant and the scenario presented by Sam, the Kind-hearted Magician:
First Point:
Savant – Two remaining cards are in play, one each in positions B and C. Magic – Two remaining cards are in play, one each in positions B and C.
Second Point:
Savant – One of the two cards still in play is the Ace and one is the Joker. Magic -- One of the two cards still in play is the Ace and one is the Joker.
Point Three:
Savant – No one changes the positions of cards B and C from the moment they are laid on the table. Magic -- No one changes the positions of cards B and C from the moment they are laid on the table.
Point 4:
Savant – The Dupes can choose either card B or pick card C. Presented as “sticking with their original choice” or “switching their choice”.
Magic -- The Dupes can choose either card B or pick card C. Presented as leaving the Yellow Star on card B or moving it to card C.
Now, think carefully, are there any differences in the real physical universe situations presented above? All three points above are apparently identical in the physical world.
Marilyn vos Savant has been declared, without doubt, to be correct in her classical presentation and answer: The Dupes should switch their choice – doing so doubles their chances of winning – if they switch they win 2/3 of the time and if the stick they win 1/3 of the time. However, Sam, our Kind-hearted Magician, shows us that if he magically erases the past in which the Dupes chose one card out of three, he creates the exactly the same two-card situation as in Savant’s presentation, but the Dupes now magically have an equal chance of winning or losing when forced to make the exact same choice. There is no longer any advantage in switching or not switching.
The ONLY thing that has changed are the words of the presentations of the scenarios – or some idea about it – but nothing in the physical world changed at all. Same series of steps, same cards in the same positions, same choices to be made. But, when the Dupes get down to making the fateful choice to “stick or switch”, the odds of winning in the two scenarios end up being quite different.
What has happened here?
Questions:
Q4: Was Marilyn vos Savant wrong after all?
Q5: Is Sam’s wand really magical and, after it has been waved over the card table, we find it has actually changed the probabilities? One Last Question:
Q6: Has there been some Sleight of Mind? If so, what was it and who performed it?
Subscribe or donate to support this site and its wholly independent host using credit card or PayPal click here