I hope you’ll forgive this minor repetition, my friends. But since the world has not yet converted to the truth, shouted by de Finetti and handful of others, that probability does not exist, and therefore nothing has a probability, it is my duty to press on.
At the least, this truth is badly needed in physics, in which quantum mechanics is mired in an instrumentalist set of beliefs that many have mistaken for Reality. As briefly as possible, a model may make good, even excellent predictions, but this does not prove that the model’s premises accurately describe Reality. Ptolemy’s epicycles are all the argument we need here.
Some of you might recall my dad and I made a coin-flip machine last fall, consisting of a clothespin, some nails, and a chunk of two-by-four. We made a video demonstrating the beast. At last count, it was up to almost a dozen views, or whatever. Is this viral?
Point of the video was to prove that coin flips do not have probabilities. The video met that burden because with every flip the coin came up heads. And continues to. (I tweaked it a bit so that it was more stable. How? By hitting my thumb into the nails.)
In other words, given the operations of the machine and coin and flip, the probability of a head is 1. This probability, like all probabilities, is conditional on the information given or assumed. There is no probability in the coin, or in the flip, or in anything.
That’s it. That’s all of probability. Simple.
Alas, you still hear talk of “data generating mechanisms” and “true distributions” and “parameter estimation” and on and on. So our work is not done.
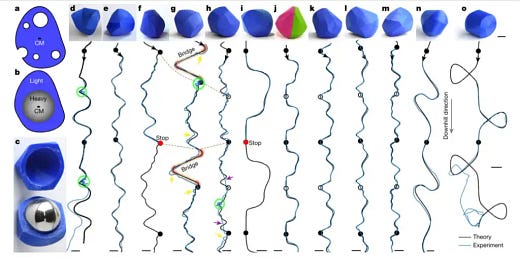
There are bright spots, though, which form the excuse for today’s post. Take this article from Popular Mechanics: Scientists Figured Out How to Design Dice to Roll Any Way You Want.
Dice throws don’t have probabilities either. Dice outcomes have causes. And these guys, like the coin guys we’ll meet below, have figured out some of the causes. Here’s the barest details we need to get the flavor of this. Their paper can be found in the link.
Scientist Yaroslav Sobolev at the Institute for Basic Science in Ulsan, South Korea—along with his colleagues—have designed an algorithm that creates wonky-shaped objects called “trajectoids” that mathematically travel along any set path. The results of the study were recently published in the journal Nature…
“For any path, you can always find such a sphere, of some radius, that when it completes two periods of the path, it will restore its 3D orientation perfectly,” Sobolev tells New Scientist. “This allows you to make a particle that will roll forever downhill, always tracing the path again and again.”
The algorithm works by tracing a moldable sphere’s contact points with the ground as it travels a predetermined path. The team then created a 3D printed shell to cover the hard metal interior and tested the results against the mathematically designed path. The trajectoids followed the designed path and even successfully repeated the path twice in most cases. (If you’re a 3D printing aficionado, you can download the trajectoid algorithm and try it out for yourself.)
So far this is all abstract. It works in the math, but they haven’t shown it in real life. The reason is simple: the oddly shaped dice have to be tossed on real surfaces. Just as our coin had to land on a real surface, which we jury-rigged as a soft pad to absorb the forces of a fall, those dice guys would have to figure what the surface does to the roll.
Even so, they have nailed the key fact, which is that dice tosses have causes, not probabilities.
Finally we come to this piece, from Smithsonian Magazine: “Gamblers Take Note: The Odds in a Coin Flip Aren’t Quite 50/50: And the odds of spinning a penny are even more skewed in one direction, but which way?”
Their paper is also at the link.
Diaconis is a professor of mathematics and statistics at Stanford University and, formerly, a professional magician. While his claim to fame is determining how many times a deck of cards must be shuffled in order to give a mathematically random result (it’s either five or seven, depending on your criteria), he’s also dabbled in the world of coin games. What he and his fellow researchers discovered (here’s a PDF of their paper) is that most games of chance involving coins aren’t as even as you’d think. For example, even the 50/50 coin toss really isn’t 50/50 — it’s closer to 51/49, biased toward whatever side was up when the coin was thrown into the air.
But more incredibly, as reported by Science News, spinning a penny, in this case one with the Lincoln Memorial on the back, gives even more pronounced odds — the penny will land tails side up roughly 80 percent of the time. The reason: the side with Lincoln’s head on it is a bit heavier than the flip side, causing the coin’s center of mass to lie slightly toward heads. The spinning coin tends to fall toward the heavier side more often, leading to a pronounced number of extra “tails” results when it finally comes to rest.
Persi was for a time one of my PhD advisors when he was at Cornell. We shared an interest in amateur magic.
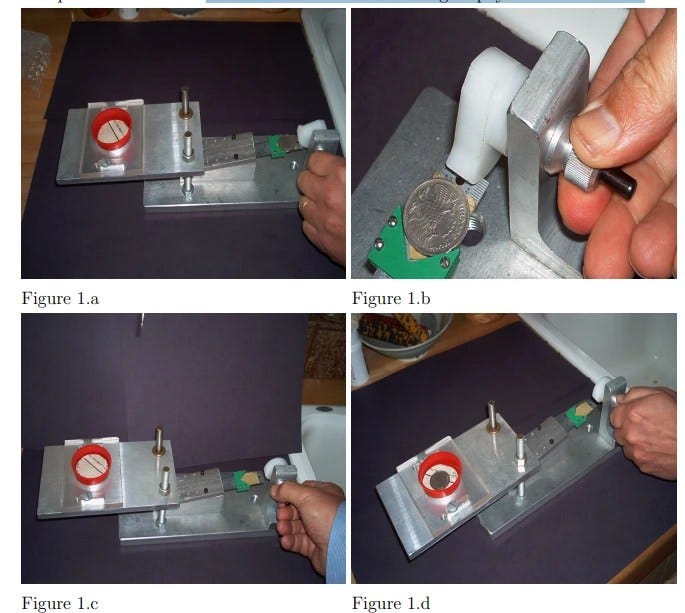
He also built a coin-tossing machine (way before I did, which is where I got the idea). Here is a picture:
A tad better than mine.
But we share the same conclusion: “We conclude that coin-tossing is ‘physics’ not ‘random’.”
We’ll save quantum mechanics for another day.
Subscribe or donate to support this site and its wholly independent host using credit card click here. Or use the paid subscription at Substack. Cash App: $WilliamMBriggs. For Zelle, use my email: matt@wmbriggs.com, and please include yours so I know who to thank.




Please do quantum mechanics next!
It may be my engineering brain that gives the ability to know that all things being equal, they're never quite perfectly equal. Murphy was an optimist, sh1t happens and when the sh1t flies it sticks to the engineer. So given that there will undoubtably be variations in the coin, toss, mech, air density, air movement, center mass, surface, alloy, density, gravitational anomalies, ect.
The solution is straight forward,
- it's always better to overbuild with redundancy.
(Dam the accountants when they say something will never happen; If it can, it will!)